
Fall 2023 Update: The Topology and Geometry Seminar has moved to this webpage.
Time: Tuesdays 1:00 - 2:15 PM, unless otherwise noted.
Location: CL 251
Most talks are held in person. Some talks are on Zoom:
https://uregina-ca.zoom.us/j/99127226830?pwd=bnFQR1R3UUdyWUxqSS9JMExMRlZwZz09
In this learning seminar, we will study topological quantum field theories (TQFTs), cobordism categories, and the cobordism hypothesis.
The lecture series will be interspersed with guest research talks, which are posted on our Youtube channel.
| Date | Speaker | Title and abstract | References |
|---|---|---|---|
| January 17 | No seminar. | ||
| January 24 | Martin Frankland | Introduction to the cobordism hypothesis Topological quantum field theories (TQFTs) are one of several mathematical approaches to quantum field theory in physics. They were introduced by Atiyah in the 1980s. The cobordism hypothesis, proposed by Baez and Dolan in the 1990s, is a statement about a classification of TQFTs. It was proved by Hopkins and Lurie, with contributions from others. I will present an overview of the cobordism hypothesis and related notions: cobordisms, TQFTs, low-dimensional examples, higher categories of cobordisms, and extended TQFTs. After the overview, I will introduce Atiyah's axioms for a TQFT. |
A pleasantry |
| January 31 On Zoom |
Igor Sikora (Bilkent University) | $RO(C_2)$-graded coefficients of $C_2$-Eilenberg-MacLane spectra In non-equivariant topology, the ordinary homology of a point is described by the dimension axiom and is quite simple - namely, it is concentrated in degree zero. The situation in $G$-equivariant topology is different. This is because Bredon homology - the equivariant counterpart of ordinary homology - is naturally graded over $RO(G)$, the ring of $G$-representations. Whereas the equivariant dimension axiom describes the part of the Bredon homology of a point graded over trivial representations, it does not put any requirements on the rest of the grading - in which the homology may be quite complicated. The $RO(G)$-graded Bredon homology theories are represented by $G$-Eilenberg-MacLane spectra, and thus the Bredon homology of a point is the same as coefficients of these spectra. During the talk, I will present the method of computing the $RO(C_2)$-graded coefficients of $C_2$-Eilenberg-MacLane spectra based on the Tate square. As demonstrated by Greenlees, the Tate square gives an algorithmic approach to computing the coefficients of equivariant spectra. In the talk, we will discuss how to use this method to obtain the $RO(C_2)$-graded coefficients of a $C_2$-Eilenberg-MacLane spectrum as a $RO(C_2)$-graded abelian group. We will also present the multiplicative structure of the $C_2$-Eilenberg-MacLane spectrum associated to the Burnside Mackey functor. Time permitting, we will further discuss how to use this knowledge to derive a multiplicative structure for the coefficients for any ring Mackey functor. |
Recording |
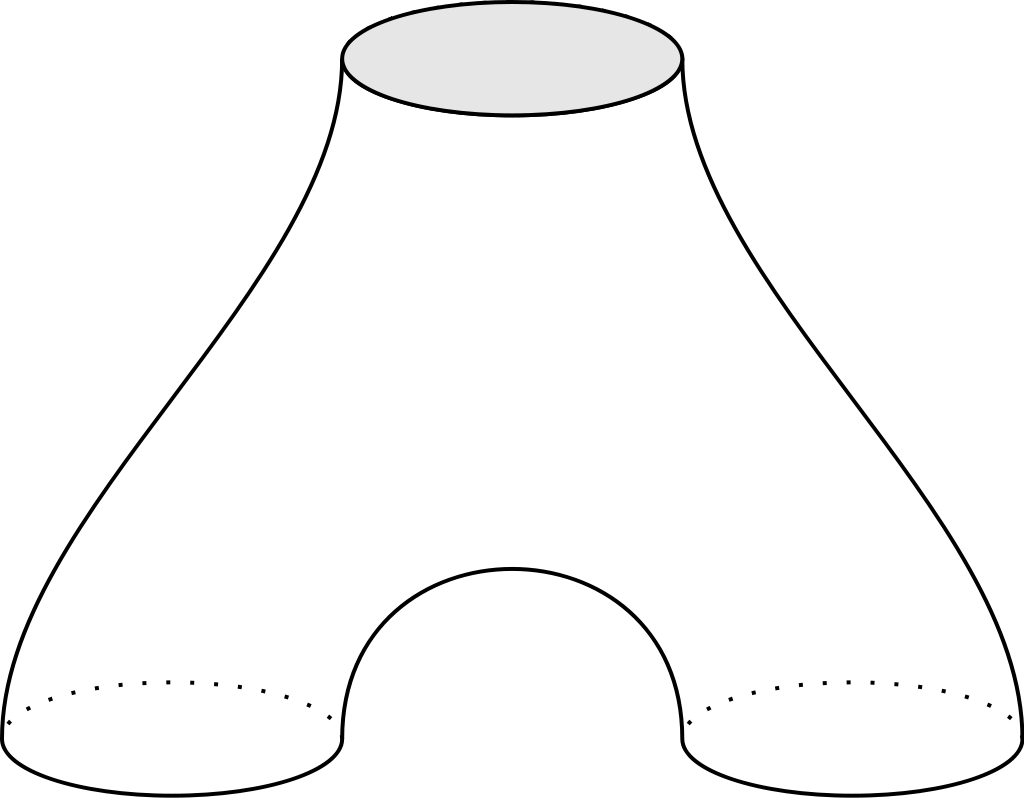
| February 7 | Carlos Gabriel Valenzuela | On the classification of 1-dimensional TQFTs In general Topological Quantum Field Theories are very diverse, but we may focus on low dimensional cases. As a starting point, we'll give a complete classification of 1-dimensional TQFTs. To this end, I'll present a review of dualization in a symmetric monoidal category, discuss a few examples focusing on the category of vector spaces, and finally present a proof for the equivalence of 1-dimensional TQFTs and finite dimensional vector spaces. |
|
| February 14 | Arnaud Ngopnang Ngompe | Classification of 2-dimensional TQFTs In this talk, we give a description of finite-dimensional commutative Frobenius algebras and we recall the description of 2-dimensional TQFTs. We show that these two structures are equivalent to each other. |
|
| February 21 | Reading Week. No seminar. | ||
| February 28 | Allen Herman | Frobenius algebras In Arnaud's talk, we saw that the 2-dimensional TQFT category is equivalent to the category of commutative Frobenius algebras with isomorphisms. In this sequel to Arnaud's talk, we take a closer look at Frobenius algebras. We will explore their algebraic and coalgebraic properties, look at important sources of examples, and see how the natural coalgebraic structure of a Frobenius algebra compares to that of bialgebras and Hopf algebras. |
|
| March 7 | Matthew Alexander | TQFTs from physical systems When the same topic appears in both mathematics and physics, there are often stark differences between how that topic is conceptualized and studied in each discipline. In this talk we will examine what it means to a physicist for a field theory to be 'topological' and how to translate that information into the more familiar language of a functor on a cobordism category. In particular we will see how TQFTs are natural models for describing quantum gravity in low dimensions. |
|
| March 14 | Pi Day. No seminar. | ||
| March 21 | Manak Singh | K-theory as a generalized cohomology theory Topological K-theory is an assignment of algebraic data to a topological space. It is a homotopy invariant and satisfies the Eilenberg-Steenrod axioms for a generalized cohomology theory. In this talk I give a rapid introduction to vector bundles over compact Hausdorff spaces. I show how this helps generate useful algebraic data associated to a space, and how the resulting assignment takes the shape of a cohomology theory. |
|
| March 28 | Martin Frankland | Extended TQFTs and higher categories The classification of 2-dimensional TQFTs was obtained by cutting up surfaces into disks, cylinders, and pairs of pants. In higher dimension, such a decomposition of n-manifolds is not available. We need to cut up manifolds not only along codimension 1 submanifolds, but also strata of codimension 2 or higher. Those refined cutting rules lead to the notion of an extended TQFT. I will sketch some attempts at making this notion precise, and how the data can be described using higher categories. |
|
| April 4 | Manak Singh | The Cobordism Hypothesis The Cobordism Hypothesis, as proposed by Baez and Dolan in 1995, provides a classification of n-extended Topological Quantum Field Theories (TQFTs). A n-extended TQFT is a symmetric monoidal n-functor from the n-category of cobordisms to an arbitrary symmetric monoidal n-category $\mathcal{C}$. The hypothesis asserts that n-extended TQFTs correspond to fully dualizable objects in $\mathcal{C}$. In 2008, Lurie outlined a proof for a more general statement, which implies the Baez & Dolan version as a consequence. In Martin's talk we encountered the motivation for defining 2-extended TQFTs. I extend on this and show why it is natural to contemplate n-extended TQFTs. I discuss Lurie's $(\infty,n)$-categorical version of the hypothesis and talk about what it means for an object to be fully dualizable in an $(\infty,n)$-category. |
|
| April 11 On Zoom |
Brandon Doherty (Stockholm University) | Cubical models of higher categories without connections Cubical sets with connections model $(\infty,1)$-categories via the cubical Joyal model structure, constructed and shown to be equivalent to the Joyal model structure on simplicial sets by Doherty-Kapulkin-Lindsey-Sattler. In the same work, an analogous model structure was constructed on the category of cubical sets without connections (i.e., having only faces and degeneracies), but was not shown to be equivalent to any other model of $(\infty,1)$-categories. In this talk, we will review the cubical Joyal model structure on cubical sets without connections and discuss the proof that it is Quillen equivalent to the Joyal model structure on simplicial sets, using a construction by which a fibrant cubical set without connections can be equipped with connections via lifting. This talk is based on the paper of the same title. |
arXiv:2207.03636 Recording |
| August 22 3:30 PM Room RIC 208 and on Zoom |
Sacha Ikonicoff (University of Ottawa) | Quillen-Barr-Beck cohomology of divided power algebras over an operad Divided power algebras are algebras equipped with additional monomial operations. They are fairly ubiquitous in the positive characteristic setting, and appear notably in the study of simplicial algebras, in crystalline cohomology, and in deformation theory. An operad is a device that encodes operations: there is an operad for associative algebras, one for commutative algebras, for Lie algebras, Poisson algebras, and so on. Each operad then comes with an associated category of algebras, and also with a category of divided power algebras. The aim of this talk is to show how André-Quillen cohomology generalises to several categories of algebras using the notion of operad. We will introduce modules and derivations, but also representing objects for modules - known as the universal enveloping algebra - and for derivations - known as the module of Kähler differentials - which will allow us to build an analogue of the cotangent complex. We will see how these notions allow us to recover known cohomology theories on many categories of algebras, while they provide somewhat exotic new notions when applied to divided power algebras. This is joint work with Martin Frankland and Ioannis Dokas. |
Recording |

For more information, contact Martin Frankland.